Magnetic fields play an important role in astrophysical accretion discs, and in the interstellar and intergalactic medium. They drive jets, suppress fragmentation in star-forming clouds and can have a significant impact on the accretion rate of stars. However, the exact amplification mechanisms of cosmic magnetic fields remain relatively poorly understood. Here I start by reviewing recent advances in the numerical and theoretical modelling of the turbulent dynamo, which may explain the origin of galactic and inter-galactic magnetic fields. While dynamo action was previously investigated in great detail for incompressible plasmas, I here place particular emphasis on highly compressible astrophysical plasmas, which are characterised by strong density fluctuations and shocks, such as the interstellar medium. I find that dynamo action works not only in subsonic plasmas, but also in highly supersonic, compressible plasmas, as well as for low and high magnetic Prandtl numbers. I further present new numerical simulations from which I determine the growth of the turbulent (un-ordered) magnetic field component (B_turb) in the presence of weak and strong guide fields (B_0). I vary B_0 over 5 orders of magnitude and find that the dependence of B_turb on B_0 is relatively weak, and can be explained with a simple theoretical model in which the turbulence provides the energy to amplify B_turb. Finally, I discuss some important implications of magnetic fields for the structure of accretion discs, the launching of jets, and the star formation rate of interstellar clouds.
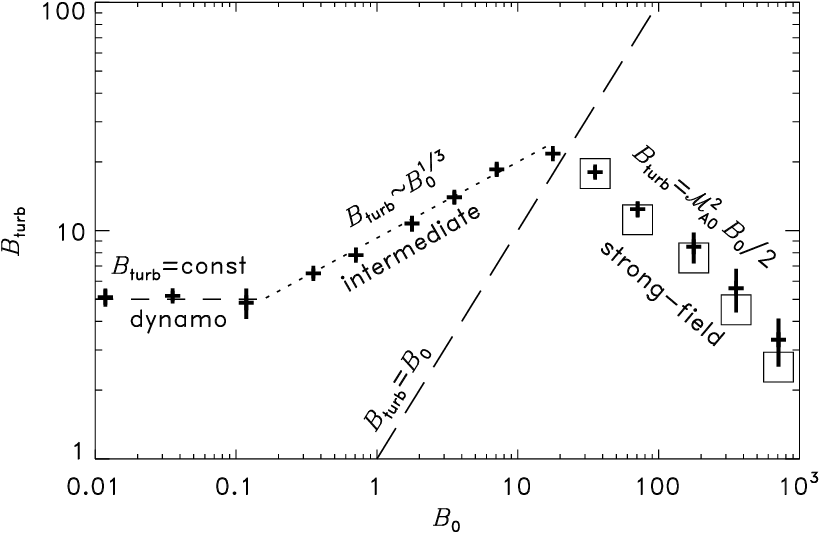
The Figure shows the turbulent (un-ordered) magnetic field (B_turb) as a function of the (ordered) magnetic guide field (B_0) the simulations. We can distinguish three regimes: i) the dynamo regime for weak B_0, ii) the intermediate regime, and iii) the strong guide-field regime. The dashed and dotted lines indicate fits of B_turb for the dynamo regime and the intermediate regime, respectively. The boxes show our new theoretical model of B_turb in the strong-field regime (see paper for details). The long-dashed line shows B_turb = B_0, which separates the intermediate from the strong-field regime.
The author thanks the two anonymous referees for their comments, which helped to improve this work. The author gratefully acknowledges funding provided by the Australian Research Council's Discovery Projects (grant DP150104329). The simulations presented in this work used high performance computing resources provided by the Leibniz Rechenzentrum and the Gauss Centre for Supercomputing (grants pr32lo, pr48pi and GCS Large-scale project 10391), the Partnership for Advanced Computing in Europe (PRACE grant pr89mu), the Australian National Computational Infrastructure (grant ek9), and the Pawsey Supercomputing Centre with funding from the Australian Government and the Government of Western Australia, in the framework of the National Computational Merit Allocation Scheme and the ANU Allocation Scheme. The simulation software FLASH was in part developed by the DOE-supported Flash Center for Computational Science at the University of Chicago.